分类： 2024

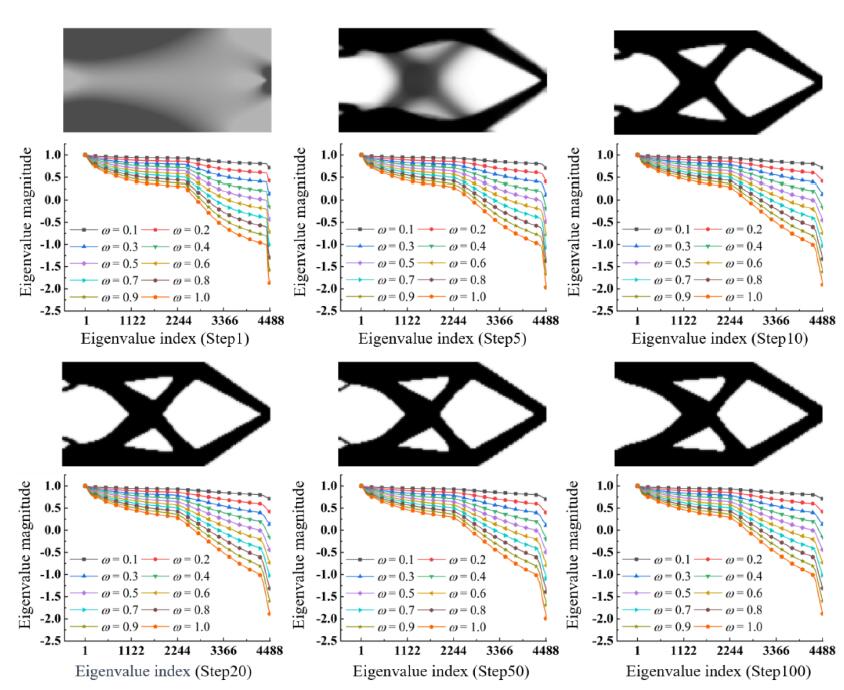
The efficiency of solving sparse linear equations in isogeometric topology optimization (ITO) can be improved by the multigrid algorithm due to its excellent convergence rate. However, its convergence rate heavily relies on the smoother’s parameters. To address this problem, a new h-refinement multigrid conjugate gradient method with adaptive damped Jacobi (ADJ-hMGCG) has been developed. By analyzing the eigenvalues of the stiffness matrix, the damping coefficient of the smoother that achieves the fastest convergence rate has been determined. Due to the significant computational resources required to compute eigenvalues in the stiffness matrix, this paper also presents a preconditioned power method based on ITO and geometric multigrid characteristics to improve the efficiency of adaptive damping solutions. The results of 2D and 3D numerical examples show that the ADJhMGCG method successfully improves the solution speed and robustness while meeting the accuracy requirements of topology optimization, and the total computational cost can be reduced by up to 59 % compared to traditional solvers for large-scale problems.

In engineering applications, plenty of components are subjected to variable-amplitude cyclic loading, resulting in fatigue damage, which is one of the main forms of structural damage. While the linear damage rule has long served as a fundamental approach, its limitations necessitate advancements for more accurate fatigue life predictions. Hence, this paper introduces a pioneering method termed nonlinear fatigue damage constrained topology optimization (NFDCTO). This method integrates several key components: the rainflow counting method to evaluate the non-proportional cyclic load levels, Basquin’s equation to describe the S-N curve, and Morrow’s plastic work interaction rule to calculate the nonlinear cumulative damage of the structure. Consequently, we establish a mathematical model for the NFDCTO method based on these components. The incorporation of penalized damage aggregation with the P-Normal function is used to address significant constraints and singularity challenges. Furthermore, by employing the adjoint method, we derive sensitivity equations for both the objective function and fatigue constraint function concerning the design variables. Subsequently, the superiority of the NFDCTO method over traditional linear fatigue damage method were verified applying 2D L-shaped beam and simply supported structures as examples. Concurrently, bridge structures were employed to investigate the effect of the sensitivity index of the material to the history of variable amplitude stresses on the optimization results. In addition, the influence of the fatigue penalty factor on the topology optimization results was assessed using a 2D cantilever beam. Finally, we verify the effectiveness and feasibility of the NFDCTO method in addressing fatigue optimization challenges for 3D structures utilizing two 3D examples.

Aiming to address the challenge of inaccurately describing the curve boundary of the complex design domain in traditional finite element mesh, this paper proposes an improved polygon mesh generation and polygon scaled boundary finite element method (PSBFEM) using non-uniform rational B-spline (NURBS) boundary. In the improved mesh generation scheme, the domain boundary will be accurately described using NURBS curves. Within this framework, a NURBS updating strategy is proposed, allowing the NURBS curve information on the boundary to be updated as the mesh changes. By employing point inversion and knot insertion, additional control points are introduced to ensure that some coincide with the nodes of the elements, thereby guaranteeing the accuracy of subsequent analyses. The boundary elements can be discretized into NURBS elements and conventional elements using SBFEM, whose physical fields are respectively constructed using NURBS basis functions and Lagrange shape functions in the circumferential direction. In the radial direction, by transforming a system of partial differential equations into a system of ordinary differential equations, which can be analytically solved without fundamental solutions. Furthermore, the internal elements can be solved directly with the traditional polygon SBFEM. The numerical examples demonstrate that the proposed method can achieve a high-quality polygon mesh with NURBS updating. Moreover, it effectively solves the corresponding polygon elements and significantly improves the accuracy of the displacement and stress solutions compared to the traditional polygon SBFEM.

Specific structures that demand to maintain the shape of a local region under external loads, such as the injection surfaces of injection molded machine formwork structures, the outer surfaces of wings and engine blades, require homogeneous local deformation. Therefore, a topology optimization method that minimizes local relative displacement differences with stress constraints for such local area is proposed in this paper. The method presents a novel topology optimization model that employs the sum of squares of the discrepancies between local displacements and their mean values as the objective function. Additionally, it adopts a P-Normal aggregation function to handle global stress constraints effectively. Sensitive equations for the objective and stress constraint functions are derived via concomitant variables. Then, the algorithmic procedure of the proposed method is illustrated and validated with 2D and 3D examples by comparing the results with those of compliance minimization. The results demonstrate that the proposed method has obvious superiority and potential application value in the minimization of local relative displacement differences. Finally, the applicability and practicality of the proposed method in the field of engineering structures is verified by structural optimization of an engineering model in injection molding equipment.

The traditional finite element analysis for irregular design domains often encounters challenges such as intricate
mesh discretization and inaccurate boundary description. In this paper, we propose a quadtree scaled boundary
finite element method based on NURBS curves where the boundaries can be accurately represented. Quadtree
decomposition, which satisfies the 2:1 rule, is employed to rapidly subdivide the analysis domain. The scaled
boundary finite element method (SBFEM) is utilized to analyze the internal elements and address the
displacement incompatibility issue of hanging nodes in the quadtree. Furthermore, the boundary element is
discretized into boundary curves and internal lines, whose displacement fields are respectively constructed by
the NURBS shape functions and the Lagrange shape functions, and then the subsequent analysis of the boundary
element is completed by SBFEM. Finally, numerical examples are tested to demonstrate the feasibility of the
proposed method, which effectively enhances computational efficiency and accuracy in solving irregular design
domains.